National Science Foundation
National Curve Bank

Diprotic Acid-Base Equilibria

Two Protons in Action!

Deposit #28

William A. Hoffman

The graphics in this deposit were created using Maple software


and were suggested to the NCB by
Jason Schattman, Ph.D.
Applications Marketing Manager
Waterloo Maple, Inc.
Background for the student . . . . .

Diprotic acids are substances (molecules) that have two protons that can be released in water.  A very common diprotic acid is sulfuric acid,  H2SO4. In water, both protons of sulfuric acid are released, giving hydrated protons 2H+(aq)  and sulfate dianion, SO4 (2-):

The pH of the solution, since it is very acidic, is low.  (The number is well below zero!)

Carbonated water (club soda) is another example of a diprotic acid.  This diprotic acid and its monoprotic acid partner are formed when carbon dioxide is bubbled through water.

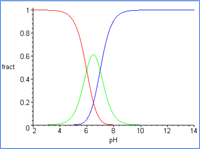
In a general case as the pH of an aqueous solution increases, a diprotic acid will ionize first to a monoprotic acid ( ha- ) and one proton ( H+ ) and then the monoprotic acid ( ha- ) will ionize to a dianion ( a= ) and liberate the second proton ( H+ ).
Study the graph below.  Think about what is happening.   As one curve decreases, what is happening to the other curve(s)?   Many students find it easier to remember a reaction if he or she actually makes a sketch of the graph. 

As the pH increases (horizontal axis), the fraction of the diprotic acid ( yellow curve )  decreases while the fraction of the monoprotic anion  ( green curve )  increases.  Then, above pH = 8, the dianion  ( red curve representing no protons ) forms as the monoprotic acid (green curve) is depleted.

Study the curves on the left (below) and then click on the graph to see the animation.  These exercises use examples of acids found in chemical and biochemical systems.

k1  =  10-6
k2  =  varies

This animation demonstrates what happens when the value of  k2 changes relative to k1.

Changing values of k1, k2   - or the pH range -   show the effect these variables have on the fraction of each species present.  Even when  k2  =  k1  (unlikely)  there is a small amount of  (ha-) present.
In this animation, study the bar moving across the graph.
k1  =  10-6
k2  =  10-10

The  pH  "bar" is animated by half -pH units which allows one to follow more accurately the fraction of diprotic  (h2a), monoprotic  (ha-), and aprotic  (a=)  species present at each pH value.
k1  = 10-9.... 10-1     (varies)
k2  = 10-14.... 10-6   (varies)

The 3d animation of k1, k2, and pH allows an overview of the way fractions of the species  (h2a),  (ha-),  and  (a=)  predominate at different pH levels.  When  pH > 9, ( a=)  will predominate unless  k2  approaches  10-14.  Conversely, at  pH  =  0, if  k1  =  10-1, the fraction of h2a is still less than 1.  Under most aqueous solution conditions, the  (ha-)  fraction will be significant.  Note the limits placed on k1 and k2 in this illustration.
Dr. Arnold O. Beckman, Inventor of the pH Meter

Any National Curve Bank deposit on acid-base reactions would not be complete without acknowledging Dr. Arnold O. Beckman, the inventor of the pH meter and a major contributor to the technology revolution of the 20th century.
 

This photo of Dr.Beckman was taken on his 100th birthday, April 10, 2000, at Casa Pacifica, San Clemente, California.  In addition to his many other accomplishments - scholar, inventor, civic leader and philanthropist - he is one of the U.S.'s oldest living Marines. 

Arnold has several rules for living a good life.  One is always do your best.  Never do anything half-heartedly.
Zaven Karian at Denison University in Granville, Ohio brought together faculty from across liberal arts departments to see what they might do with computational tools using Maple software.  Hoffman's  pH  animations are one outcome.  The project was supported by the Fund for Improvement of Post Secondary Education (FIPSE) of the Department of Education (Grant #P116B30079).

The NCB thanks Hoffman and Karian for their fine efforts.  Moreover, we thank Jason Schattman of Waterloo Maple, Inc. for calling this work to our attention.  This deposit represents a merger of applied mathematics with chemistry using computer software.
For the Maple code that created the animations please see  < http://www.mapleapps.com/categories/science/chemistry/html/Ab6.html  >