|
Power
Point
Slide Show
References
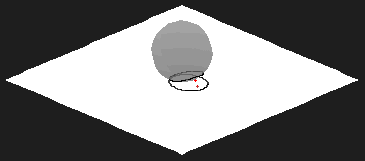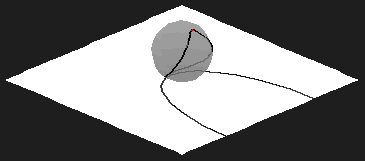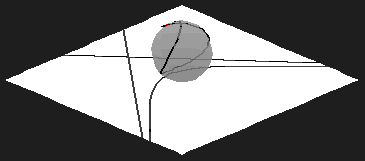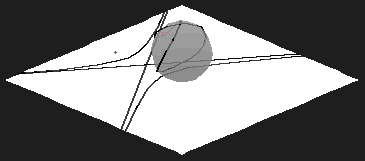
Johannes Kepler said, “A parabola is an
ellipse with a focus at infinity.” On its
face, this statement is
nonsense. What does it mean for a focus to be
“at” a point that is not
defined? In this animation, points on the
plane are associated with
points on a sphere by stereographic
projection. The north pole of the
sphere corresponds to the point at infinity.
This is the one point
compactification of the plane. Curves in the
plane correspond to curves
on the sphere. In particular, a family of
ellipses in the plane with
one focus that tends to infinity is displayed.
On the sphere, when the
focus is at the point at infinity, the curve
corresponds exactly to a
parabola in the plane. The focus on the sphere
is then sent beyond
infinity, over the top. The corresponding
curves in the plane are
hyperbolas. In this sense, hyperbolas are
ellipses where one focus has
been sent to infinity and then back from the
other side, just as Kepler
described. Using the one point
compactification, we can make sense of
Kepler’s descriptions of the conic sections.
|
For more
animations please see
< http://jgroah.nhmccd.cc/index.htm
>.
For more on Kepler please see
< ..//birthdayindex/dec/dec27kepler/dec27kepler.htm
>.
|
Note:
There are many spellings of the name of Pavel
Sergeiivich Aleksandrov
(1896-1982) as it must be translated from the
Cyrillic
alphabet. In researching this material
we found Alexandroff
and Alexandrov
as well as Aleksandrov.
Aleksandrov
is used by Gardner,
Katz and Weisstein.
| Howard
Eves, An Introduction to the History of
Mathematics, 6th ed., Saunders
College Publishing, p. 324. |
R. J.
Gardner, "Geometric Tomography." Not. Amer.
Math. Society, 42,
422-429, 1995.
|
Victor
J. Katz, A History of Mathematics,
2nd ed., Addison Wesley Longman, 1998,
p. 832.
Katz
writes
of the chain of famous names
associated with the birth of
Algebraic Topology.
Noether, Aleksandrov,
Eilenberg and Mac Lane
are
all joined in this whole
new 20th century field
of study.
"When in the
course of our
lextures (in
Göttingen in 1926
and 1927) she first
became acquainted
with a
systematic
construction of
combinatorial
topology, she
immediagely
observed that it
would be worthwhile
to study directly
the groups of
algebraic complexes
and cycles of a
given polyhedron and
the subgroup
of the cycle group
consisting of cycles
homologous to zero
...."
Aleksandrov's
memorial to Emmy
Noether
(1882-1935)
|
|
|
Eric W.
Weisstein, CRC Concise Encyclopedia
of Mathematics, CRC Press,
1999.
|
|
|
Kepler
introduced the word "focus" into the
geometry of conics. Indeed,
it has been written that if the Greeks had
not perused the conics,
Kepler could not have superseded
Ptolemy. But he is primarily
remembered for his three laws, a cornerstone
of astronomy.

Kepler
is honored on many stamps.
|
"I
am writing a book for my
contemporaries - or does
not matter - for
posterity. It may be
that my book will wait for
a hundred years
for a
reader. Has not God
waited 6,000 years for an
observer?"
Johannes
Kepler, Harmony
of the Worlds,
1619
|
|
|

Both Brahe
and Kepler are honored in Prague . . . .
|

and for a time, both
lived on this same street. |
|