|
J. B.
Thoo
Manjinder
S. Bains
____________
Thoo and
Bains created the
images in our
animation using Octave,
a high-level
langauge primarily
intended for
numerical
computations. This
free software is
mostly compatible
with Matlab.
Open
this link to
learn more about
Octave.
|
As a young
man, William Neile
interacted closely
with his far more
famous mentor, John
Wallis.
Neile was
educated at Wadham
College, Oxford, one
of the newest in the
17th century.
Recently Sir Roger
Penrose retired there.
Cardan was
primarily a physician
but made other
significant
contributions in
addition to
mathematics.
His "cardanic
suspension," a device
used to hold a compass
stable while sailing on
the high seas, appears
on a postage stamp.
|
Tartaglia's stellated
truncated hexahedron from his 1543
edition of Euclid's "Elements."
|
|
|
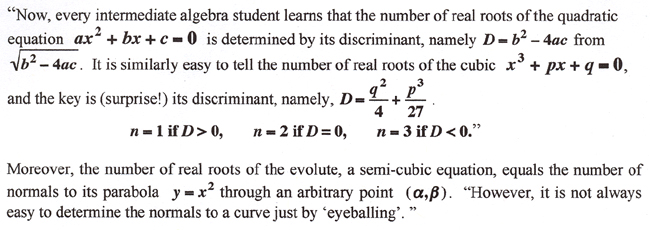
Did
you know . . .
Please note the second degree term is
missing. Moreover, neither Tartaglia nor
Cardan knew of negative and/or imaginary
solutons to equations. However, Cardan
was the first to notice a cubic might have
three roots and wrote the negative solutions
were "fictitious."
|
A brief list of
references that should be in most
university libraries.
|
From
the authors of Deposit # 80 . . .
Bains, Manjinder S. and
J. B. Thoo , The Normals to a Parabola and
the Real Roots of a Cubic, The College
Mathematics Journal, 38 (4)
September 2007, pp. 272-277. |
Gray, Alfred, Modern
Differential Geometry of Curves and
Surfaces with MATHEMATICA®,
CRC Press, 1998, p. 1027.
|
| Lockwood, E. H., A Book of
Plane Curves, Cambridge University
Press, 1961, pp. 167-171. |
| Weisstein, E. W., CRC
Concise Encyclopedia of Mathematics,
CRC Press, 1999, pp. 589 - 590. See Evolute.
|
|
