National Science Foundation
National Curve Bank

The Nephroid Family of Curves

 
    Jakob Bernoulli
(a.k.a. James, Jacque, Jacob)
For Nephroids on the right. . . .
    
Replay the animation of Freeth's Nephroid
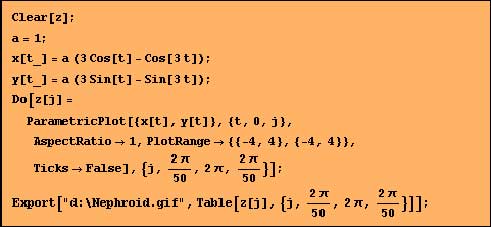
An epicycloid of two cusps traces a classic nephroid.

Play the animation
MATHEMATICA®Code


For the student . . . .
In addition to MATHEMATICA@, the Nephroid family of curves is easily entered and modified on a graphing calculator.  
Historical Sketch
The name  nephroid means "kidney-shaped" from the Greek for nephróros meaning "kidney" and éidos meaning form.

The nephroid was investigated extensively in the late 17th century.    Earlier, an exchange of ideas among scholars centered on circles and cycloids.   Mersenne, Galileo, Roberval, Descartes, Pascal, and Wallis among others, contributed to the discussion.

Their successors naturally went on to identify more complicated plane curves with unique properties.   For example, in 1692 Jakob Bernoulli  (1654 - 1705) showed that when a light source is placed at the cusp of a cardioid, the catacaustic, or envelope of the family of reflected rays, is a nephroid.  

"Catacaustics of a circle can be seen as the bright curves on the surface of coffee in a cup or upon the table inside a curcular napkin ring."  (You can) "observe some catacaustics of a circle using a cup of liquid and a movable light source."
Howard Eves  
Some of the more important names:
  • Nephroid Evolute
  • Nephroid Involute
  • Catacaustic of a Circle
  • Catacaustic of a Cardioid
  • Spherical Nephroid
    		•  Some of the more important investigators:
  • Huygens  (1678)
  • Tschirnhausen  (ca. 1679)
  • Jakob Bernoulli  (1692)
  • Daniel Bernoulli  (1725)
  • Proctor  (1878)
  • Freeth  (1879)
    		•
    Some of the more important historical publications:
  • Huygens' Traité de la luminère published in 1690.
    		•
  • T. J. Freeth's paper published by the London Mathematical Society in 1879.
    		•
  • R. A. Proctor, The Geometry of Cycloids (London, 1878).
    		•
    A useful catalog of curves can be found in older editions of the Encyclopaedia Britannica.  Search under Curves, and/or Special Curves written by R. C. Archibald.
    Useful Links and Books
    http://www-history.mcs.st-and.ac.uk/history/Curves/Nephroid.html
    http://mathworld.wolfram.com/Nephroid.html
    Eves, Howard, An Introduction to the History of Mathematics, 6th ed,. The Saunders College Publishing, 1990.
    Gray, Alfred,  Modern Differential Geometry of Curves and Surfaces with MATHEMATICA®, 2nd ed., CRC Press, 1998,  p. 898.
    Lockwood, E. H., A Book of Curves, Cambridge University Press, 1961.
    Shikin, Eugene V., Handbook and Atlas of Curves, CRC Press, 1995.
    Yates, Robert,  CURVES AND THEIR PROPERTIES, The National Council of Teachers of Mathematics, 1952.
    MATHEMATICA® Code and animations contributed by
    Gus Gordillo, 2004.