The Gaussian Distribution

The Gaussian Distribution, also called the Frequency Curve, Bell Curve, or Normal Distribution, is one of the most widely studied topics in all mathematics. Two of the most common variations of the equations are . . .

As a probability function:

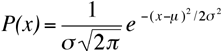
Probability Function Equation

As the so-called Standard Normal Distribution:

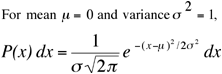
The Deutsche Mark note with Gauss' picture and his hallmark distribution curve has been replaced in circulation by the Euro.

The strength of the Gaussian Distribution is that it is often a very good approximation. This assumption is based on the Central Limit Theorem studied in the Calculus. The "CLT" proves that the mean of any data set, with a distribution having both a finite mean and finite variance, tends to be Gaussian. This implies that test scores, height, weight, etc., when graphed will tend to have a "bell" shape, with very few at either the high or low end.

From Roger Thatcher's address to the BSHM Christmas meeting on December 13, 2003:

Re: Thatcher's employment as a statistician in the Ministry of Labour or Department of Employment.

"I made some analyses of the earning statistics and eventually produced two papers for the Royal Statistical Society. One interesting result concerned the earnings of manual men. These followed a lognormal distribution. That is to say, the logarithm of their earnings has a normal distribution, which is now sometimes called the Bell curve (though earlier it was known as the Gaussian distribution, originally discovered by de Moivre). There had been earlier surveys of manual earnings at various dates right back to 1886 and these had been lognormal too. What was extremely unexpected, though, was that these lognormals all had almost exactly the same variance. This meant that although the level of earnings had changed enormously since 1886 in terms of £ per week, the percentage differences between higher paid and lower paid workers had hardly changed at all. I remember that one day a whizz-kid from Downing Street came round to tell us that there was going to be a brand new incomes policy, which this time would change the distribution of earnings. I said: 'Well, it is a lognormal distribution. What shape do you want it to be?' "

References

< ..//famouscurves/famous.htm#FrequencyCurve >

Click here for a view of the spread of a Normal Distribution.

Weisstein, Eric W., CRC Concide Encyclopedia of Mathematics, CRC Press, 1999, p. 716.

For Mathematica® code that will create many variations of this curve see Gray, A., MODERN DIFFERENTIAL GEOMETRY of Curves and Surfaces with Mathematica®, 2nd. ed., CRC Press, 1998, pp. 393-394.